E. Parameter dependence of the Action

We consider the action integral (24). Expanding the integrand we get

$$x \tanh ^{-1} \left\{ \frac{x \sqrt{x ^{4} - 4 \vert \Th... ...{1}{2 \vert \Theta \vert} x ^{3} + {\mathcal{O}} (x ^{4}) ;$$

Then the upper integration limit, 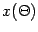$x (\Theta)$, can be expanded as

$$x ( \Theta ) = \Theta + {\mathcal{O}} ( \Theta ^{2})$$

so that when both expansions hold, we can write

$$\bar{S} (\Theta) \sim \frac{11 \vert \Theta \vert ^{3}}{12} + {\mathcal{O}} (\Theta ^{4}) .$$

We thus see that the leading term for small $\vert \Theta \vert$ is $\sim \vert \Theta ^{3} \vert$.